Abstract: In signal analysis, we generally intercept finite waveform data for Fourier transform. This truncation process will cause leakage, resulting in power spreading to the entire spectrum, generating a large amount of "haze data" and failing to obtain correct spectral results. . Although it is known that windowing can suppress leakage, complex window function expressions and abstract mainlobe sidelobe description methods are more confusing. Let us abandon the formula to introduce the selection of window functions in an easy-to-understand way.
Windowing and window function
In the digital signal processing, there are common rectangular window, Hanning window, Hamming window and flat top window. The expression of window function is not repeated here. Only the use of window function is discussed. The following figure visually describes the signal windowing. The basic features of the process and window functions.
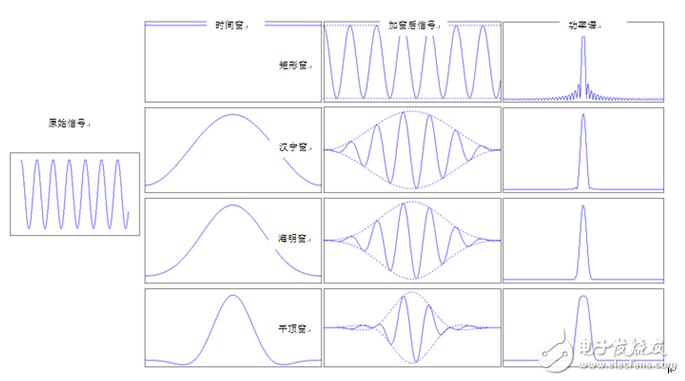
Figure 1 Frequency map after signal windowing
Intuitively, in the time domain, windowing is actually a window function as a modulated wave, and the input signal is used as a carrier for amplitude modulation (referred to as amplitude modulation). The rectangular window does not change the waveform in the time window intercepted, that is, only the truncated signal is output as it is. The other three window functions modulate the signal at the beginning and end of the time window to zero.
More generally, most window function shapes have shapes that gradually fall from the middle to the sides, but differ in detail such as the speed of the drop. This feature embodies the purpose of windowing—reducing leakage caused by truncation. All window functions reduce the extra spectrum produced by sudden changes in the signal at the truncated edge by reducing the amplitude of the signal at the start and end.
2. Selection of window function
It is obvious from Fig. 1 that the time domain of the signal changes significantly after windowing. Since the subsequent processing is generally performed by Fourier transform, we mainly analyze the effect of windowing on the Fourier transform result. The main features of the Fourier transform are frequency, amplitude and phase, and the effect of windowing on the phase is linear, so it is generally not considered. The effects on frequency and amplitude are discussed below.
The effect of windowing on frequency and amplitude is related. First, you need to remember a conclusion: for a single frequency signal in the time domain, the spectrum after windowing is to shift the peak position of the window spectrum to the frequency of the signal, and then proceed. Vertical zoom. The effect of windowing depends on the power spectrum of the window. Combined with the power spectrum of the last column function in Figure 1, it is easy to understand the description of the main lobe, side lobes, etc. of the window features commonly seen in other introductory articles.
Looking at the power spectrum of the window function, from top to bottom, the main peak of the window function (ie, the main lobe) is getting thicker and thicker, and the side peaks (ie, side lobes) on both sides are getting less and less. The name of the flat top window is also due to the main lobe. The peak is flat and named. The main lobe width may be superimposed on the spectrum of the nearby frequency, which means that it is more difficult to find the largest frequency point in the superposed power spectrum, that is, the frequency resolution is lowered, and it is difficult to locate the center frequency. More side lobes mean more signal power leakage, the main lobe is weakened, that is, the amplitude accuracy is reduced.
With the law, the use of window functions is much simpler. When the frequency resolution is high, a window with few side lobes, such as a Hanning window, is used, and there are too many side lobes of the rectangular window, and the leakage is too large to suppress the leakage; when the amplitude is required to be accurate, a flat top window can be used. Of course, for a transient signal or a shock waveform whose process time is less than the window, the start and end of the signal itself is zero, there is no leakage caused by the truncation, and no window suppression is required, so only a rectangular window is needed. For continuous periodic waveforms, different windows can be combined to achieve the results of interest.
Note: Can you design a perfect window function, only the main lobe has no side lobes, and the main lobe is narrow to only one column? the answer is negative. The narrow main lobe and the side lobes are like the ends of the fascia. The next time it is pressed, the other side will be lifted, which is irreconcilable.
Freestanding Gas Cookers
Enjoy the control and convenience of gas cooking with Freestanding Gas Cookers, featuring four gas burners and 4 brass burner cap to help you create delicious meals for your family and friends.
Key Features
Fuelled by natural gas, Freestanding Cooker is economical and delivers delicious results, letting you enjoy tender, moist roasts with lower running costs.
The spacious cooktop features a triple ring burner for fast, efficient heating, and flame failure protection to cut off the gas flow if the flame is extinguished.
The gas oven offers a range of cooking functions, including electric grill, static gas, and fan assisted gas for even heat distribution.
Crafted with low porosity titanium enamel and a removable glass door, the Cooker is easy to clean.
With a 10 AMP plug already fitted to the Cooker, installation to a standard power outlet is simple and easy.
Black color body
Glass top
Stainless Working Top
4 Gas Burners with 4 brass burner cap
(1 large 2 medium 1 small)
Manual Ignition
Enamel Grill
Knobs with Base
Double Glass Oven Door (mirror)
Downside Oven Burner
1 Pcs Oven Grill
Aluminum Handle
Duck feet
Oven Size:60L
Product Size:50X50X81cm

Freestanding Gas Cookers
Freestanding Gas Cookers,Free Standing Electric Combination Cooker Oven,Large Volume Oven Cooker,Six Burners Glass Cover Cooker
xunda science&technology group co.ltd , https://www.gasstove.be
